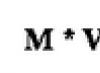In this lesson, we will repeat the main provisions of the theory and solve more complex problems on the topic "Parallelism of lines and planes."
At the beginning of the lesson, let's recall the definition of a straight line, parallel to a plane and the theorem-a sign of parallelism of a straight line and a plane. We also recall the definition of parallel planes and the theorem-sign of parallel planes. Next, we recall the definition of skew lines and the theorem-attribute of skew lines, as well as the theorem that through any of the skew lines it is possible to draw a plane parallel to another line. We draw a conclusion from this theorem - the statement that two skew lines correspond to a single pair of parallel planes.
Next, we solve several more complex problems using the repeated theory.
Topic: Parallelism of lines and planes
Lesson: Repetition of the theory. Solving more complex problems on the topic "Parallelism of lines and planes"
In this lesson, we will repeat the main provisions of the theory and solve more complex problems on the topic "Parallelism of lines and planes".
Definition. A line and a plane are called parallel if they have no common points.
If a line not lying in a given plane is parallel to some line lying in this plane, then it is parallel to the given plane.
Let a straight line be given a and plane (Fig. 1). There is a straight line in the plane b, which is parallel to the line a. From parallel lines a and b parallel line follows a and planes. 

1. Geometry. Grade 10-11: a textbook for students of educational institutions (basic and profile levels) / I. M. Smirnova, V. A. Smirnov. - 5th edition, corrected and supplemented - M.: Mnemozina, 2008. - 288 p.: ill.
Assignments 9, 10 p. 23
2. Three lines intersect in pairs. Can any plane be parallel to all these lines?
3. Through the point M, only one straight line can be drawn, parallel to the planes α and β. Are these planes parallel?
4. Two trapeziums have a common midline. The α plane passes through the smaller bases of the trapezium, and the β plane passes through the larger bases of the trapezium. Are planes α and β parallel?
5. ABCD- quadrilateral. The point M lies outside its plane. Do the midpoints of the segments lie in the same plane? MA, MV, MS, MD?
For two straight lines in space, four cases are possible:
Straight lines match;
The lines are parallel (but not the same);
The lines intersect;
The lines intersect, i.e. do not have common points and are not parallel.
Consider two ways of describing lines: canonical equations and general equations. Let the lines L 1 and L 2 be given by the canonical equations:
L 1: (x - x 1) / l 1 = (y - y 1) / m 1 = (z - z 1) / n 1 , L 2: (x - x 2) / l 2 = (y - y 2) / m 2 \u003d (z - z 2) / n 2 (6.9)
For each straight line from its canonical equations, we immediately determine a point on it M 1 (x 1 ; y 1 ; z 1) ∈ L 1 , M 2 (x 2 ; y 2 ; z 2) ∈ L 2 and the coordinates of the direction vectors s 1 = (l 1 ; m 1 ; n 1 ) for L 1 , s 2 = (l 2 ; m 2 ; n 2 ) for L 2 .
If the lines coincide or are parallel, then their direction vectors s 1 and s 2 are collinear, which is equivalent to the equality of the ratios of the coordinates of these vectors:
l 1 / l 2 \u003d m 1 / m 2 \u003d n 1 / n 2. (6.10)
If the lines coincide, then the direction vectors are also collinear with the vector M 1 M 2 :
(x 2 - x 1) / l 1 \u003d (y 2 - y 1) / m 1 \u003d (z 2 - z 1) / n 1. (6.11)
This double equality also means that the point M 2 belongs to the line L 1 . Therefore, the condition for the lines to coincide is the fulfillment of equalities (6.10) and (6.11) simultaneously.
If the lines intersect or cross, then their direction vectors are non-collinear, i.e. condition (6.10) is violated. The intersecting lines lie in the same plane and, therefore, vectors s 1 , s 2 and M 1 M 2 are coplanarthird order determinant composed of their coordinates (see 3.2):
Condition (6.12) is satisfied in three cases out of four, since for Δ ≠ 0 the lines do not belong to the same plane and therefore intersect.
Let's bring all the conditions together:

The mutual arrangement of the lines is characterized by the number of solutions for the system (6.13). If the lines coincide, then the system has infinitely many solutions. If the lines intersect, then this system has a unique solution. There are no direct solutions in the case of parallel or crossing direct solutions. The last two cases can be separated by finding the direction vectors of the lines. To do this, it suffices to calculate two vector works n 1 × n 2 and n 3 × n 4 , where n i = (A i ; B i ; C i ), i = 1, 2, 3.4. If the resulting vectors are collinear, then the given lines are parallel. Otherwise they are interbreeding.
Example 6.4.

The directing vector s 1 of the straight line L 1 is found by the canonical equations of this straight line: s 1 = (1; 3; -2). The directing vector s 2 of the straight line L 2 is calculated using the vector product of the normal vectors of the planes, the intersection of which it is:

Since s 1 \u003d -s 2, then the lines are parallel or coincide. Let us find out which of these situations is realized for given lines. To do this, we substitute the coordinates of the point M 0 (1; 2; -1) ∈ L 1 into the general equations of the line L 2 . For the first of them, we obtain 1 = 0. Therefore, the point M 0 does not belong to the line L 2 and the lines under consideration are parallel.
Angle between lines. The angle between two lines can be found using direction vectors direct. The acute angle between the lines is equal to the angle between their direction vectors (Fig. 6.5) or is complementary to it if the angle between the direction vectors is obtuse. Thus, if for the lines L 1 and L 2 their direction vectors s x and s 2 are known, then the acute angle φ between these lines is determined through the scalar product:
cosφ = |S 1 S 2 |/|S 1 ||S 2 |

For example, let s i = (l i ; m i ; n i ), i = 1, 2. Using formulas (2.9) and (2.14) to calculate vector length and the scalar product in coordinates, we get

Chapter IV. Lines and planes in space. Polyhedra
§ 46. Mutual arrangement of lines in space
In space, two distinct lines may or may not lie in the same plane. Let's consider the corresponding examples.
Let the points A, B, C do not lie on one straight line. Draw a plane through them R and choose some point S that does not belong to the plane R(Fig. 130).
Then the lines AB and BC lie in the same plane, namely, in the plane R, lines AS and CB do not lie in the same plane. Indeed, if they lay in the same plane, then the points A, B, C, S would also lie in this plane, which is impossible, since S does not lie in the plane passing through the points A, B, C.
Two distinct lines that lie in the same plane and do not intersect are called parallel. Coinciding lines are also called parallel. If straight 1 1 and 1 2 parallel, then write 1 1 || 1 2 .
In this way, 1
1 || 1
2 if, first, there is a plane R such that
1
1 R and 1
2 R and second, or 1
1 1
2 = or 1
1 = 1
2 .
Two lines that do not lie in the same plane are called intersecting lines. Obviously, skew lines do not intersect and are not parallel.
Let us prove one important property of parallel lines, which is called the transitivity of parallelism.
Theorem. If two lines are parallel to a third, then they are parallel to each other.
Let 1 1 || 1 2 and 1 2 || 1 3 . We need to prove that 1 1 || 1 3
If straight 1 1 , 1 2 , 1 3 lie in the same plane, then this statement is proved in planimetry. We will assume that the lines 1 1 , 1 2 , 1 3 do not lie in the same plane.
Through straight lines 1 1 and 1 2 draw a plane R 1 , and through 1 2 and 1 3 - plane R 2 (Fig. 131).

Note that the straight line 1
3 contains at least one point M that does not belong to the plane
R 1 .
Draw a plane through the line and the point M R 3 that intersects with the plane R 2 along some line l. Let's prove that l coincides with 1 3 . We will prove the "method by contradiction".
Let's assume that the line 1
does not match the line 1
3 . Then 1
crosses the line 1
2 at some point A. This implies that the plane R 3 passes through point A R 1 and straight 1
1 R 1
and therefore coincides with the plane R one . This conclusion contradicts the fact that the point M R 3 does not belong to the plane R 1 .
Therefore, our assumption is wrong, and therefore 1
= 1
3 .
Thus, it is proved that the lines 1 1 and 1 3 lie in the same plane R 3 . Let us prove that the lines 1 1 and 1 3 do not intersect.
Indeed, if 1 1 and 1 3 intersect, for example, at point B, then the plane R 2 would pass through a straight line 1 2 and through point B 1 1 and, therefore, would coincide with R 1 , which is impossible.
A task. Prove that angles with codirectional sides are equal.
Let the angles MAN and M 1 A 1 N 1 have co-directed sides: the ray AM is co-directed to the ray A 1 M 1, and the ray AN is co-directed to the ray A 1 N 1 (Fig. 132).

On the rays AM and A 1 M 1 we set aside segments AB and A 1 B 1 equal in length. Then
|| and |BB 1 | = |AA 1 |
as opposite sides of a parallelogram.
Similarly, on the rays AN and A 1 N 1 we set aside segments AC and A 1 C 1 equal in length. Then
|| and |CC 1 | = |AA 1 |
From the transitivity of parallelism it follows that || . And since |BB 1 | = |CC 1 | , then BB 1 C 1 C is a parallelogram, and therefore |BC| = |B 1 C 1 |.
Consequently, /\
ABC /\
A 1 B 1 C 1 and .
The video course "Get an A" includes all the topics necessary for the successful passing of the exam in mathematics by 60-65 points. Completely all tasks 1-13 of the Profile USE in mathematics. Also suitable for passing the Basic USE in mathematics. If you want to pass the exam with 90-100 points, you need to solve part 1 in 30 minutes and without mistakes!
Preparation course for the exam for grades 10-11, as well as for teachers. Everything you need to solve part 1 of the exam in mathematics (the first 12 problems) and problem 13 (trigonometry). And this is more than 70 points on the Unified State Examination, and neither a hundred-point student nor a humanist can do without them.
All the necessary theory. Quick solutions, traps and secrets of the exam. All relevant tasks of part 1 from the Bank of FIPI tasks have been analyzed. The course fully complies with the requirements of the USE-2018.
The course contains 5 large topics, 2.5 hours each. Each topic is given from scratch, simply and clearly.
Hundreds of exam tasks. Text problems and probability theory. Simple and easy to remember problem solving algorithms. Geometry. Theory, reference material, analysis of all types of USE tasks. Stereometry. Cunning tricks for solving, useful cheat sheets, development of spatial imagination. Trigonometry from scratch - to task 13. Understanding instead of cramming. Visual explanation of complex concepts. Algebra. Roots, powers and logarithms, function and derivative. Base for solving complex problems of the 2nd part of the exam.
The lines lie in the same plane. if they 1) intersect; 2) are parallel.
For the belonging of the lines L 1: and L 2: to the same plane so that the vectors M 1 M 2 \u003d (x 2 -x 1; y 2 -y 1; z 2 -z 1), q 1 =(l 1 ;m 1 ;n 1 ) and q 2 =(l 2 ;m 2 ;n 2 ) were coplanar. That is, by the condition of the complanarity of three vectors, the mixed product M 1 M 2 s 1 s 2 =Δ==0 (8)
Because the condition of parallelism of two lines has the form: , then for the intersection of lines L 1 and L 2 , so that they satisfy condition (8) and so that at least one of the proportions is violated.
Example. Explore the relative position of the lines:
Direction vector of the straight line L 1 – q 1 =(1;3;-2). Line L 2 is defined as the intersection of 2 planes α 1: x-y-z+1=0; α 2: x+y+2z-2=0. Because line L 2 lies in both planes, then it, and hence its direction vector, is perpendicular to the normals n 1 and n 2 . Therefore, the direction vector s 2 is the cross product of vectors n 1 and n 2 , i.e. q 2 =n 1 X n 2 ==-i-3j+2k.
That. s 1 =-s 2 , means the lines are either parallel or coincide.
To check whether the lines coincide, we substitute the coordinates of the point M 0 (1;2;-1)L 1 into the general equations L 2: 1-2+2+1=0 - incorrect equalities, i.e. point M 0 L 2,
so the lines are parallel.
The distance from a point to a line.
The distance from the point M 1 (x 1; y 1; z 1) to the line L given by the canonical equation L: can be calculated using the cross product.
 It follows from the canonical equation of the straight line that the point M 0 (x 0; y 0; z 0) L, and the directing vector of the straight line q=(l;m;n)
It follows from the canonical equation of the straight line that the point M 0 (x 0; y 0; z 0) L, and the directing vector of the straight line q=(l;m;n)
Let's build a parallelogram on vectors q and M 0 M 1 . Then the distance from the point M 1 to the line L is equal to the height h of this parallelogram. Because S=| q x M 0 M 1 |=h| q|, then
h= ![]() (9)
(9)
The distance between two straight lines in space.
L 1: and L 2:
1) L 1 L 2 .
d= 
2) L 1 and L 2 - crossing
d= 
Mutual arrangement of a straight line and a plane in space.
For the location of a straight line and a plane in space, 3 cases are possible:
line and plane intersect at one point;
line and plane are parallel;
the line lies in a plane.
Let the straight line be given by its canonical equation, and the plane - by the general
α: Ax+By+Cz+D=0
The straight line equations give the point M 0 (x 0; y 0; z 0) L and the direction vector q=(l;m;n), and the plane equation is a normal vector n=(A;B;C).
1. Intersection of a line and a plane.
If a line and a plane intersect, then the direction vector of the line q not parallel to the plane α, and therefore not orthogonal to the normal vector of the plane n. Those. their dot product nq≠0 or, in terms of their coordinates,
Am+Bn+Cp≠0 (10)
Determine the coordinates of the point M - points of intersection of the line L and the plane α.
Let's move from the canonical equation of the straight line to the parametric one: , tR
We substitute these relations into the equation of the plane
A(x 0 +lt)+B(y 0 +mt)+C(z 0 +nt)+D=0
A,B,C,D,l,m,n,x 0 ,y 0 ,z 0 are known, let's find the parameter t:
t(Al+Bm+Cn)= -D-Ax 0 -By 0 -Cz 0
if Am+Bn+Cp≠0, then the equation has a unique solution that determines the coordinates of the point M:
t M = -→ (11)
The angle between a line and a plane. Conditions of parallelism and perpendicularity.
Angle φ between line L :
with guide vector q=(l;m;n) and plane
: Ax+By+Cz+D=0 with normal vector n=(A;B;C) ranges from 0˚ (in case of parallel line and plane) to 90˚ (in case of perpendicularity of line and plane). (Angle between vector q and its projection onto the plane α).
– angle between vectors q and n.
Because the angle between the line L and the plane is complementary to the angle , then sin φ=sin(-)=cos =- (the absolute value is considered because the angle φ is acute sin φ=sin(-) or sin φ =sin(+) depending on the direction of the straight line L)